11/1/2019: I'm spending my sabbatical at the Corcoran School of the Arts & Design, now part of GWU. 
The Corcoran School was founded in 1878 in the Corcoran Gallery of Art in Washington, DC, (more or less across the street from the White House). The Corcorn Gallery was founded by businessman William Wilson Corcoran in 1869 as a public museum to exhibit his private art collection (this was his second museum. His first museum, 2 blocks away is now the Smithsonian Renwick Gallery). The Corcoran Gallery existed for over150 years as an independent art museum in Washington, DC. The art school became part of GWU in 2014, and the museum is slated to be a partnership between GWU and the National Gallery of Art. Here is the stairs to my office "studio", and a picture showing its fantastic skylight,
The goal of my sabbatical is to study digital and mathematical arts. I was inspired by various projects I have been involved in over the years: a math exhibit at the Maryland Science Center in Baltimore 25 years ago (see this, this and this), the Math and Art courses that I have taught about five times at GWU and the Corcoran, some my research, which involves such visually exciting mathematical objects as tilings and fractals, and by the review I wrote for the Bulletin of the AMS on the Man Ray & Hiroshi Sugimoto exhibits at the Phillips Collection in 2015 (the Phillips is another independent Washington, DC art museum). The Phillips exhibit was about photographs and paintings of mathematical models from the turn of the century (19th to 20th), mostly from Germany, by some very famous mathematicians, notably Felix Klein. This kind of "mathematical modeling" went out of style after WW I. But modeling is making a come-back because of various digital technologies.
During this sabbatical, my plan is to learn to use laser cutters and 3-d printers. These are located in the "Clean Fab" studio in the basement of the Corcoran (which interestingly, also has sewing machines, including a vintage Singer model from the 19th century). I am learning to use various model making software to make a lot of models (so far Mathematica, Adobe Illustrator, OpenSCAD, Meshmixer, Meshlab). I want to thank the Corcoran School, and in particular the director Kim Rice, and James Huckenpahler, an artist and professor at the Corcoran who has provided me with a lot of valuable advice.
I am now about two months into the sabbatical and I thought it was time to show some of my work.
- Laser cut of "binary tiling":
See https://tilings.math.uni-bielefeld.de/substitution/binary/
- I am using laser cutting to make all five Platonic and thirteen Archemedean solids (as of 11/4, I've made all but four). Here are the three largest Archimedean ones: the great rhombicosidodecahedron, truncated dodecahedron, and truncated icosahedron
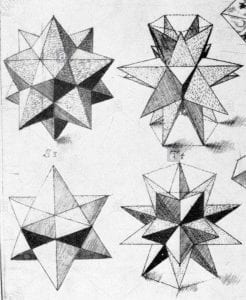
(I suppose I should not be surprised, but corrugated cardboard is very tenacious). Archimedes supposedly listed them around 230 BC in a now lost text, but the first modern listing was Kepler in his book Harmonices Mundo, 1619 - The two Kepler polyhedra: the great dodecahedron and small dodecahedron (with Kepler's drawings from Harmonices Mundo,1619)
- Here is a 3-d printed version of the great dodecahedron (left). These are challenging to make because they are not convex. Right is the laser cut great dodecahedron, a Poinsot (Kepler-Poinsot) polyhedron:
There is one more Kepler-Poinsot polyhedron, the great icosahedron. It will appear here later. - Menger sponge, and stage 1-sided along regular hexagon to reveal star-shaped hole;
- One of my projects is to 3-d print as many as possible of the models that were in the Man Ray/Hiroshi Sugimoto exhibit at the Phillips Collection. Here is my iphone photo of my print of the Clebsch surface, and Sugimoto's:
- A different version of the Clebsch surface figures in several Man Ray works:
- Man Ray's Julius Cesar (left), and (right) my 3d print. This is the real part of the essential singularity at z=0 of the function f(z)=exp(1/z). This is trying to illustrate the "chaotic" behavior near the origin that Picard's Theorem suggests (see this). Notice how the detail in the foreground of my print is muddled. I will try reprinting it later at a larger scale, and maybe editing out some of this detail (notice that it was edited out in the model Man Ray painted).
- ManRay's Anthony and Ceopatra and my model of Keun's surface.
