This animation shows the reflection/transmission on a potential step:
Items to be discussed:
- Real and imaginary part of the Gaussian wave packets move with a different speed than |Ψ(x,t)|². This Illustrates phase velocity vs. group velocity
- Once the packet hits the step (also shown in animation), parts of it are reflected, and parts are transmitted, which helps understand the respective undergraduate standard formulae. One could even position an observer at some positive x-value, that integrates |Ψ(x,t)|² over time to measure how much of the wave packet is transmitted.
- The last thought can then also be set into relation with conservation of probability and the continuity equation.
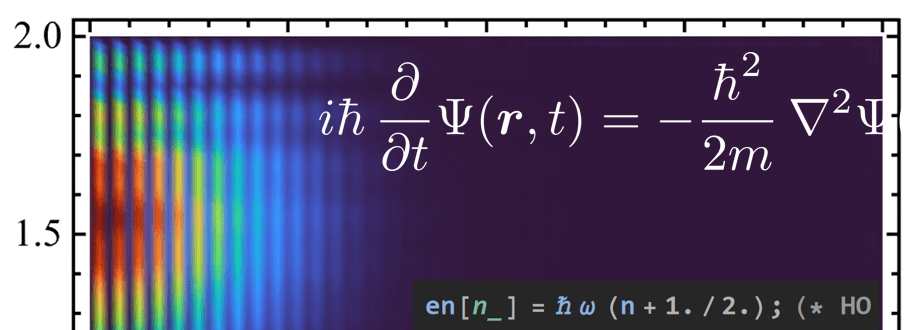
Why is the packet's phase velocity group velocity?
Thank you for your comment! The group velocity is faster, it is just a bit hard to see. There's another animation on this blog comparing group and phase velocities in more detail, for the Gaussian wave packet.
Ignore my first comment please. I was interrupted and accidentally posted. Thanks.
Ok. Thanks again for your interest!
Before contact with the potential step and after reflection, the phase group velocity. What has happened to switch from anomalous to normal dispersion? By the way, this would seem to contradict DeBroglie. Thanks.
The other example is named "Gaussian wave packet in free space".
Hello !
I think the reflected wave should have the same wavelength as the incident wave. Am I wrong ?
Hi Sergio,
Thanks for stopping by! as you can see I don''t get many comments;) The underlying reflected wave (thin solid and dashed lines) does have the same wave length as in incoming one. The overall wave packet, though (thick solid red line), gets spread out over time, so the reflected wave packet is indeed wider than the incident wave packet. Is that your observation?